What Does
Hydraulic Radius Mean?
Hydraulic radius, R, a measure of channel flow efficiency, is defined as the ratio of the cross-sectional area of fluid flow, A, to the length of the wetted perimeter, P.
R=AP
The hydraulic radius is one of the main properties that control the amount of fluid discharge of a channel and its ability to move sediments. A high hydraulic radius value indicates that the channel contains a lower volume of contact fluid and a greater cross-sectional area.
These conditions result in increased flow velocity and capacity, as well as improved channel efficiency.
Another term sometimes used for this quantity is hydraulic mean depth.
Trenchlesspedia Explains Hydraulic Radius
The hydraulic radius formula applies to differing channel shapes, including rectangular, trapezoidal, and circular. The hydraulic radius is a direct function of the shape of its conduit. For example, for circular cross-sections, such as sewers and pipe culverts, the hydraulic radius is not half the diameter, as the name implies. The hydraulic radius, in this case, is one quarter the diameter of a full pipe.
The complexity of hydraulic radius calculations varies according to the shape of the channel being evaluated, with the rectangular channel being most simplistic. Estimates for the other channel shapes and a partially filled pipe are not as straightforward.
Rectangular Channel
Consider a rectangular channel with the depth of flow denoted by the symbol, y, and the channel width represented by b, as shown below.
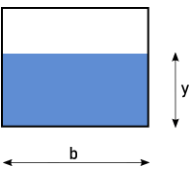
Figure 1: Rectangular Channel with Depth, y, and Width, b (source)
The area of flow, A, for this channel would be:
A=by
The wetted perimeter, P takes into consideration all surfaces exposed to the fluid:
P=b+y+y or P=2y+b
Applying the hydraulic radius equation to this scenario shows that for a rectangular channel:
R=AP= by2y+b
Trapezoidal Channels
Trapezoidal channels commonly form the basis for natural channel design, although some human-made waterways are of this shape. The calculation of the hydraulic radius for trapezoidal channels, in addition to the liquid depth, y and bottom width, b, considers the width of the liquid surface, B and the slope value, z. The slope value is the gradient of the non-parallel sides of the trapezoid.
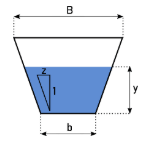
Figure 2: Trapezoidal Channel (source)
For a channel of this nature, A is found as follows:
A=y×B+b2 or A=by+y2z
For the perimeter, Pythagoras’ Theorem is applied giving the perimeter, P, as:
P=b+2y1+z2
Thus, the hydraulic radius would be:
R=by+y2zb+2y1+z2
Circular Pipe Filled with Liquid
Similarly, to a rectangular channel, calculating the hydraulic radius of a circular pipe that is filled is straightforward, as it involves the application of the area and perimeter of a circle.
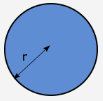
Figure 3: Circular Channel Completely Filled (source)
Area of Circle, A = r2
Perimeter of Circle, P = 2πr
Hydraulic radius, R = r22πr = r2
Circular Pipe Partially Filled
In sewer pipe design, scenarios in which the pipe is not fully filled need to be considered. For a circular pipe that is somewhat filled, parameters such as arc length and circle segment area come into play. The diagrams below illustrate the considerations for this scenario.
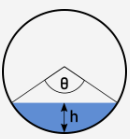

Figure 4: Circular Pipe Partially Filled (source)
In this case, the area is calculated by applying the circle segment formula.
A= r2(θ-sinθ)2
The circle shown to the left of figure 4 indicates that the wetted perimeter is equal to the arc length corresponding to the angle.
P=rθ where θ=2arccos[r-hr]
Applying the area and the perimeter equations to find the hydraulic radius gives:
R= r2(θ-sinθ)2 /rθ